Lecture 11 : Gravitational Waves

1  Introduction

Gravitational waves are predicted in General Relativity and other theories of gravity. They have never been detected directly, but in the next decade that might change, as there are presently a handful of experiments being produced around the world endevouring to find them. Indirect effects of gravity waves have been detected in the periastron advance of the binary pulsar, which we looked at in a previous lecture. When and if gravity waves are directly detected, it will open a fundamentally new window into the Universe.

Gravity waves are produced by an accelerated mass, similar to the production of light waves by an accelerated charge. It is tempting to compare them to electro-magnetic radiation: light travels through space, whereas gravity waves are oscillations in space-time itself. They are predicted to travel at, or at least close to, the speed of light. There is no practical way that gravity waves of sufficient intensity can be produced in the laboratory; such as using rapidly spinning bars or the like; all gravity wave detectors look for sources in the Universe, such as binary black holes or neutron stars, supernova explosions which form neutron stars, black hole mergers and relic gravitational radiation from the Big Bang.

The effect of gravity waves on matter are very weak. A free mass will oscillate in response to a passing gravity wave, but the amplitude of the oscillation is very small, even if the energy carried by gravity waves is quite large. This is because the resistance of space to the oscillations is very high - space acts like a very stiff material.

In the 1960's, the first efforts to detect gravitational waves experinmentally were made by Joseph Weber at the University of Maryland. An aluminium bar was set up in a lab, isolated as much as possible from surrounding disturbances, and the length of the bar monitored in the hope of detecting passing gravitational waves. Several bar detectors of this type are in operation around the world, such as 2.3 ton bar at Louisiana State University, ALLEGRO. Others are in Italy and Australia. Such bars are like radio antennae: natural modes of vibration in the bar are amplified by the gravity waves and, hopefully, detected.

The second technique to find the waves is to suspend two masses freely and monitor the distance between them. Like the bars, the masses must be isolated as best as possible from the cornucopia of surrounding disturbances, ranging from earthquakes, temperature variations, and nearby man-made and natural vibrations. The distance between the masses is monitored using interference techniques and lasers. Such detectors are called interferometers.

The most impressive of these interferometers is LIGO or the Laser Interferometer Gravitational-Wave Observatory, which is sited in Livingstone Louisiana and its twin in Hanford, Washington. The project very recently began the test phase of operation, a two year period in which its properties will need to be measured and understood, before attempts can be made at Gravitational wave detection. The two items were constructed for just over $ 200 million. Two are needed because there are huge numbers of local background disturbances - only the true gravitational events will be seen at both observatories, which are on opposite sides of the US. A third antennae, in Europe, would allow the direction of the wave to also be established, and efforts are underway to acheive this.

2  Gravity Wave Amplitude

The amount of gravitational energy released per unit time (the luminosity L) from a rotating object is given by the quadrupole formula

L ~  c5 G e2 æ è  Rs R ö ø 2   æ è  V c ö ø 6

(1)

where M is the mass and R is the mean radius of the object, the rotational velocity is V, the Schwarzschild radius is R_s = 2GM/c² and the asymmetry of the object is e. For a sphere, e = 0.

The characteristic amplitude h_c produced is

hc =  16p2 G c4  f2 D I e

(2)

where I is the moment of inertia, f is the frequency of the wave, and D is the distance. The characteristic frequency f is just the oscillation frequency of the gravitating mass. In the case of a pulsar or neutron star this is of order the rotation rate.

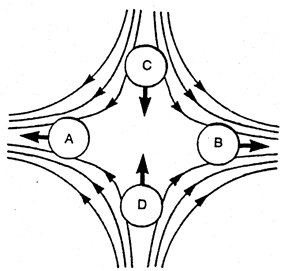
The reaction of masses in a gravitational wave train is to contract and expand perpendicular to the wave direction. This is illustrated in figure 11.1. Four masses are arranged in a cross. The gravity wave is polarised such that along one direction, expansion of the system takes place and perpendicular to this contraction takes place.

Figure 1: Reaction of four masses placed at A, B, C and D, in the plane perpendicular to the direction of propagation of a gravity wave. Source:

http://nedwww.ipac.caltech.edu/level5/ESSAYS/Boughn/boughn.html

If the masses are separated by characteristic scale L, then the change in the scale dL is of order the gravity wave amplitude h_c,

dL L ~ hc.

(3)

This quantity, dL/L is called the strain.

3  LIGO

LIGO consists of two arms, each about 4 km long, arranged in an L-shape and containing two test masses which should respond to passing waves. The tubes are about a meter wide and are evacuated - this makes about 10,000 cubic meters of vacuum! At the ends of the tubes are specially designed mirrors which reflect most of the light from a laser at the other end, but a little leaks through. The optical path length is thus increased greatly by the light moving back and forth. Light is sent down both arms by dividing it with a beam-splitter. The interference pattern where the beams combine is then used to determine if the path length down the two arms is changing, hopefully as a result of gravity waves. In phase, the light produces a bright spot, while out of phase the spot disappears.

The LIGO experiment safegaurds against false detections by having twin experiments. Gravity waves travel at or close to the speed of light, so that an event in one detector should be seen in the other with a time delay of not more than 10 ms. The event should look very similar in both detectors. The second LIGO observatory, at Hanford, will also have a half-length arm of 2 km, in which the event should be seen at half the amplitude.

What about other experiments? There are quite a few coming: an Italian/French experiment near Pisa with 3 km arms called VIRGO; a German/British collaboration GEO with 600 meter arms. In Australia a LIGO-type detector is planned, while in Japan a 300 meter arm system called TAMA has been constructed. LIGO itself had a 40 meter prototype experiment which was built and run successfully, even taking data which could be used to constrain the event rate for various types of gravitational waves. LIGO will run for several years in testing and data taking mode, and then be upgraded to a system which could be over 10 times more sensitive, in 2005. This increased sensitivity means that a volume of the Universe some 10³ times greater is probed!

4  Payoff for Physics

The detection of gravity waves has enormous potential for understanding a wide range of sources, which perhaps may never be understood from electro-magnetic radiation alone.

• Gravity waves are predicted in a number of theories of gravity, not just General Relatity. The amplitude, propgation speed and polarisation properties will allow some of these theories to be winnowed out.

• the waves are predicted to travel at the speed of light. If this is confirmed it will verify predictions of zero rest mass for the graviton.

• verify the existance of black holes and test space curvature predictions near compact objects.

• test predictions for ``in-spiralling'' of neutron stars or black holes; measure event rates; constrain star formation and supernova rates for the parent population.

• birth of neutron stars in supernova explosions; test of supernova models; spin of the new neutron star.

• star quakes on neutron stars; changes in the stars surface structure i.e. the dynamics of the ``crust'' and perhaps internal structure.

• probe beyond the ``optical'' barrier presented by the last scattering surface of the microwave background when matter recombined. Gravity waves are produced in some versions of the Big Bang theory at very early times.

Figure 2: Spectrum of predicted gravitational wave amplitudes as a function of frequency for a range of astrophysical sources.

5  Moving into space

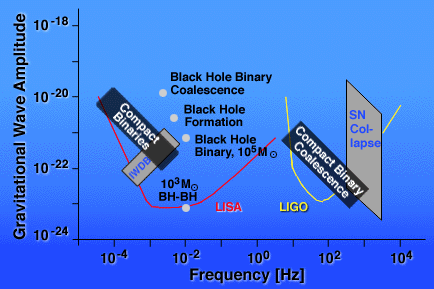
There is a fundamental limit on the frequency of detectible gravitational waves on the Earth of about 10 Hz, set by the background sources (such as the tides, weather systems, etc). The way to explore the high frequency regime, which contains a lot of interesting possible sources (see figure 11.2). The proposed mission to carry this out is LISA.

Figure 3: The LISA mission, a proposed space-borne gravitational wave detector

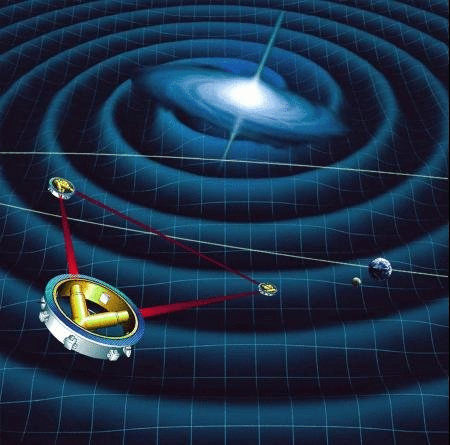
LISA consists of three ``arms'', actually three satellites in a triangle with sides of order 5 million km, sited in the ecliptic plane and about 20 degrees behind te Earth. LISA would fly in about 2008.

From the LISA website : The three LISA spacecraft flying in formation will act as a giant Michelson interferometer, measuring the distortion of space caused by passing gravitational waves. Each spacecraft will contain two free-floating 'proof masses'. The proof masses will define optical paths 5 million kilometers long, with a 60 degree angle between them. Lasers in each spacecraft will be used to measure changes in the optical path lengths with a precision of 20 picometers.

The main objective of this mission would be to learn about massive black holes and possible detect gravity waves from the early universe. Merging super massive black holes in the distant universe are another possible source. LISA would have great sensitivity to nearby neutron stars and binaries, for many of which the physics are well enough understood that the gravitational radiation spectrum can be predicted. Failure to detect such radiation would be a major surprise.

6  Sources of gravity waves

Figure 4: Three major sources of gravity waves: black hole merging, binary black holes, and much closer to home, ordinary compact binaries

6.1  Galactic Binaries

Neutron stars may generate signal if they deviate enough from perfect spheres. This matter is currently under discussion, and there are a number of proposals for maintaining assymetry on such an object, such as accretion flows or magnetic fields. Of definite interest is the merging of neutron star pairs (NS-NS merging). Presently, a number of groups are computing such events using simulations in order to estimate the gravitational flux. This is a very demanding task even for the fastest supercomputers!

Low mass BH pairs or NS-BH pairs might also generate a signal, although there are expected to be less of them.

A few cataclysmic binaries, contact binaries, interacting white dwarfs are also possible sources - especially because their positions are known, it is much easier to search for them. GAIA will create immense, accurate catalogs of such sources (including accurate distances), which will help the searchers.

6.2  Cosmic Background

Gravitational radiation from the Big Bang itself is predicted in certain models. LISA would be able to see a background ``rumble'' of gravitational waves at a level as low as 10^-9 of the closure density of the Universe, possibly at a frequency near 1 mHz. The ability to detect this will depend on the more local noise generated by nearby white dwarf binaries. More exotic phenomena, such as phase transitions in the early universe and cosmic strings might also give a signal.

6.3  Formation of massive black holes

Massive black holes are now almost certain to be present in the cores of most galaxies - the evidence coming mainly from Hubble Space Telescope and X-ray observations. M31 is an interestingcase - it shows a double nucleas at its core, which might indicate that a merger even took place and, eventually, two black holes there will also merge. Such an event would be visible in gravitational waves at huge distances. All these black holes formed at some time, with evidence indicating that they are at least active as quasars around red shifts of 2 to 4. The black holes may have formed early, from very dense regions coming out of the big bang, or they may have been built up over time, possible from many smaller black holes. Either way, gravitational radiation is expected and may be measurable with LISA.

7  Further reading

http://www.nas.edu/ssb/21fpcch3.htm

http://www.nas.edu/ssb/21fpcch4.htm

http://sci.esa.int/content/doc/45/26693_.htm

Sources:

http://lisa.jpl.nasa.gov/science/binaries.html

http://lisa.jpl.nasa.gov/science/cosmic.html

Observatories:

LIGO at http://www.ligo.caltech.edu/

VIRGO at http://www.pi.infn.it/virgo/

GEO at http://www.rzg.mpg.de/grav/home.html

TIGA at http://phwave.phys.lsu.edu/www/tiga/

References

[]

Irion, R. ``LIGO's mission of gravity'' April 2000, Science, 288, 420.

[]

Dupuis, Réjean. ``Searching for gravitational radiation from pulsars''. Thesis, 1998.

[]

Finn, L.S., 1999, ``Gravitational radiation sources and signatures'', http://xxx.lanl.gov/abs/gr-qc/9903107